Spectrum of periodic signals
In order to be able to conveniently and clearly represent the amplitude and phase of a signal at different frequencies, a representation of the spectrogram can be used.
In the Fourier analysis, the variation of the amplitude |Fn| or Cn of each component with respect to the frequency nω1 is referred to as the amplitude spectrum of the signal.
The change of the phase φn of each component with the angular frequency nω1 is called the phase spectrum of the signal.
The amplitude spectrum and the phase spectrum are collectively referred to as the spectrum of the signal.
The triangular Fourier series frequency is non-negative, the corresponding spectrum is generally called the unilateral spectrum ; the exponential form of the Fourier series frequency is the entire real axis, so it is called the bilateral spectrum .
Let's take the periodic signal function as an example to see how the Fourier level function should draw the phase spectrum and the amplitude spectrum.
periodic function:

The corresponding component magnitude of the function:
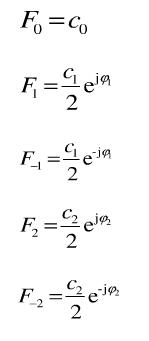
The single-sided, bilateral, phase, and amplitude spectra of the final Fourier series function are shown in the following figure:

Power X (Qingdao) Energy Technology Co., Ltd. , https://www.qdpowerxsolar.com